Q: If velocity is zero, why wouldn’t acceleration be zero as well? Seeing that both velocity and acceleration are related and given that finding the acceleration relies on the velocity, why is the acceleration unknown at a specific time instance if the velocity is known to be zero at that time?
A: Acceleration is the change rate of velocity, so the acceleration is not necessarily zero if the velocity is zero at an instant in a motion. Think about “Turning point” in a Free Fall motion as an example.
Q: I understand if you put the pivot point in the middle of the square, both forces will have zero torque. However, if you point the pivot on one of the corners, wouldn’t there be net torque so it wouldn’t be in static equilibrium?
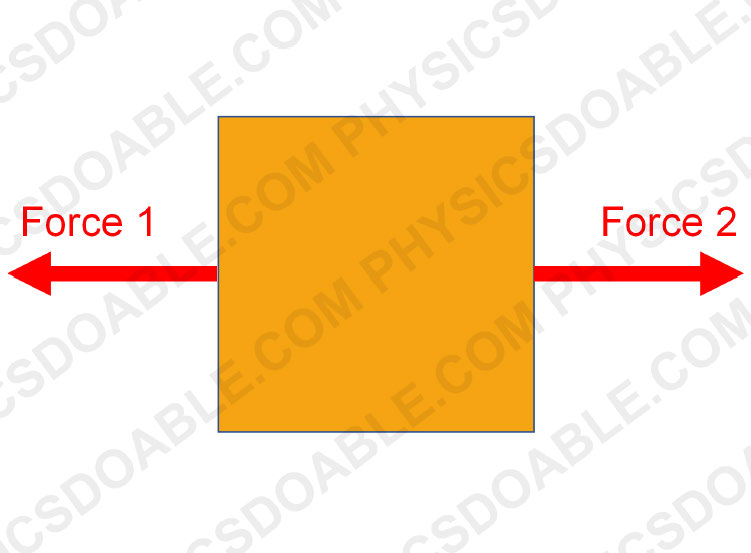
A: If you choose another point, for example, the lower left corner, as the pivot, the force on the left causes a counter-clockwise (positive) torque, while the force on the right causes a clockwise (negative) torque. The two torques equal in magnitude, but opposite in direction, so the net torque is still ZERO!
